A Rising Star in Mathematics: Young Chinese Scientist Solves Century-Old Problem
Let's Talk About Math—in Chinese!
Idiom of the week: 炸开锅 zhà kāi guō
Meaning: cause a sensation, create an uproar
华人数学家王虹和Joshua Zahl的一篇论文,在数学圈炸开了锅。A paper by Chinese mathematician Wang Hong and Joshua Zahl caused a sensation in the mathematics community.
Great News in the World of Mathematics!
Chinese mathematician Wang Hong, along with her collaborator Joshua Zahl, has published a groundbreaking 127-page paper solving a century-old mathematical mystery known as the Kakeya Conjecture. Wang Hong is now a strong contender for the prestigious Fields Medal—potentially the first Chinese recipient and only the third woman to achieve this honor!
Have you ever wondered what the smallest area an infinitely thin needle can sweep when rotated might be? Wonder no more! Let’s dive into the details in Chinese and expand our math vocabulary together.
2月26日,华人数学家王虹和Joshua Zahl的一篇论文,在数学圈炸开了锅。几何测度论中最瞩目的未解难题——Kakeya集合猜想,已在三维空间中被成功证实!多人猜测:王虹或能锁定下届菲尔兹奖。
数学圈 (shùxué quān) – mathematics community
炸开锅 (zhà kāi guō) – cause a sensation, create an uproar
几何测度论 (jǐhé cèdù lùn) – geometric measure theory
瞩目 (zhǔmù) – attract attention, draw focus, be highly notable
未解难题 (wèijiě nántí) – unsolved problem
集合 (jíhé) – set (in mathematics)
猜想 (cāixiǎng) – conjecture
三维空间 (sānwéi kōngjiān) – three-dimensional space
成功证实 (chénggōng zhèngshí) – successfully proven
锁定 (suǒdìng) – secure, lock in
下届 (xià jiè) – next session, next term, next edition (of an event or award)
菲尔兹奖 (fēi'ěrzī jiǎng) – Fields Medal
On February 26, a paper by Chinese mathematician Wang Hong and Joshua Zahl caused a sensation in the mathematics community. One of the most prominent unsolved problems in geometric measure theory—the Kakeya set conjecture—has been successfully proven in three-dimensional space! Many speculate that Wang Hong may secure the next Fields Medal.
一个困扰数学家一个多世纪的超级难题,如今被北大校友攻克了!
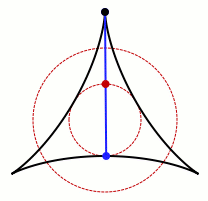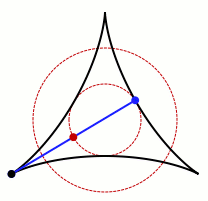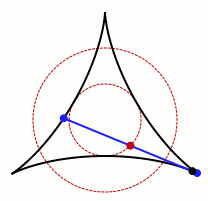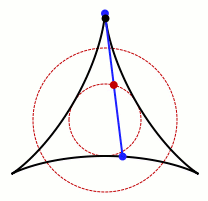
纽约大学和不列颠哥伦比亚大学数学教授联手,用一份长达127页证明,正式宣告——「Kakeya集合」猜想尘埃落定。Kakeya集合的核心问题是——如果你要在空间里「转动」一个线段,让它覆盖所有方向,最小的空间需要多大?
困扰 (kùnrǎo) – trouble, bother, perplex
校友 (xiàoyǒu) – alumnus/alumna
攻克 (gōngkè) – overcome, conquer
联手 (liánshǒu) – join forces, collaborate
长达 (chángdá) – as long as (used to emphasize length or duration)
宣告 (xuāngào) – announce, declare
尘埃落定 (chén'āi luòdìng) – settle, be concluded (literally: "the dust has settled")
核心 (héxīn) – core, central
转动 (zhuàndòng) – rotate, turn
线段 (xiànduàn) – line segment
覆盖 (fùgài) – cover, encompass
方向 (fāngxiàng) – direction
A super difficult problem that has troubled mathematicians for over a century has now been solved by a Peking University alumnus!
Mathematicians from New York University and the University of British Columbia joined forces and, with a 127-page proof, officially declared that the Kakeya set conjecture has been settled.
The core question of the Kakeya set is: If you want to "rotate" a line segment in space so that it covers all directions, what is the smallest space required?
挂谷猜想源于日本数学家挂谷宗一(Sōichi Kakeya)1917年提出的一个几何问题。数学家们早已知道,2D平面不够用,必须通过3D空间来解决问题。但关键在于,能否找到一个「超级小」的3D区域,仍让这个线段指向每个方向?
如今这个谜团,被破解了!
挂谷猜想 (Guàgǔ cāixiǎng) – Kakeya conjecture
源于 (yuányú) – originate from, stem from
几何 (jǐhé) – geometry
问题 (wèntí) – problem, question
平面 (píngmiàn) – plane (in geometry)
不够用 (bú gòu yòng) – not sufficient, not enough
仍 (réng) – still, yet
指向 (zhǐxiàng) – point to, direct toward
谜团 (mítuán) – mystery, puzzle
破解 (pòjiě) – solve, unravel
The Kakeya conjecture originates from a geometric problem proposed in 1917 by the Japanese mathematician Sōichi Kakeya. Mathematicians have long known that a 2D plane is not sufficient and that the problem must be solved in 3D space. But the key question is whether it is possible to find an extremely small 3D region that still allows the line segment to point in every direction.
Now, this mystery has been solved!
王虹和Joshua Zahl教授通过层层推导,精妙的逻辑和计算,研究了在ℝ⊃3;中具有以下性质的δ管(δ tubes)集合:即不会有太多管道被包含在同一个凸集V内。
王虹现任纽约大学库朗数学研究所(NYU Courant)数学副教授,北大数学系本科毕业;Joshua Zahl现任不列颠哥伦比亚大学数学系副教授。
他们得出,来自这样一个集合的管道的并集,必须具有几乎最大的体积。最终证明了ℝ⊃3;中的每个Kakeya集,都具有Minkowski和Hausdorff维数3。
层层推导 (céngcéng tuī dǎo) – step-by-step derivation
精妙 (jīngmiào) – exquisite, ingenious
逻辑 (luóji) – logic
计算 (jìsuàn) – calculation, computation
性质 (xìngzhì) – property, characteristic
管道 (guǎndào) – tube, pipeline
凸集 (tūjí) – convex set (mathematics)
现任 (xiànrèn) – currently serve as, hold the position of
数学系 (shùxué xì) – mathematics department
不列颠哥伦比亚大学 (Bùlièdiān Gēlúnbǐyǎ Dàxué) – University of British Columbia (UBC)
本科 (běnkē) – undergraduate degree
并集 (bìngjí) – union (in set theory)
体积 (tǐjī) – volume (of an object or space)
最终 (zuìzhōng) – ultimately, finally
维数 (wéishù) – dimension (in mathematics)
Minkowski 维数 (Minkowski wéishù) – Minkowski dimension
Hausdorff 维数 (Hausdorff wéishù) – Hausdorff dimension
Through rigorous derivations, precise logic, and calculations, Professors Wang Hong and Joshua Zahl studied a set of δ tubes in ℝ³ with the following property: not too many tubes can be contained within the same convex set V.
Wang Hong is currently an associate professor of mathematics at the Courant Institute of Mathematical Sciences at NYU and earned his undergraduate degree from Peking University's mathematics department. Joshua Zahl is an associate professor in the mathematics department at the University of British Columbia.
They concluded that the union of tubes from such a set must have almost the largest possible volume. Ultimately, they proved that every Kakeya set in ℝ³ has a Minkowski and Hausdorff dimension of 3.
一时间,全网忍不住猜测:如果这篇论文最终通过严格的同行评审,王虹极有可能成为中国首位获得菲尔兹奖的数学家,以及全球第三位拿下菲尔兹奖的女性得主。
前两位分别是,伊朗裔美国数学家Maryam Mirzakhani和乌克兰数学家Maryna Viazovska。
作为数学界至高荣耀,菲尔兹奖每4年颁发一次,只给40岁以下的数学家。
一时间 (yī shíjiān) – in no time, instantly
全网 (quán wǎng) – the entire internet
忍不住 (rěn bù zhù) – can't help but, unable to resist
猜测 (cāicè) – guess, speculate
同行评审 (tóngháng píngshěn) – peer review
极有可能 (jí yǒu kěnéng) – very likely, highly probable
首位 (shǒuwèi) – first position, first place
菲尔兹奖 (Fēi'ěrzī jiǎng) – Fields Medal
女性得主 (nǚxìng dézhǔ) – female laureate
伊朗裔美 (Yīlǎng yì Měi) – Iranian-American
乌克兰 (Wūkèlán) – Ukraine
至高荣耀 (zhìgāo róngyào) – highest honor
颁发 (bānfā) – award, present (a prize)
In no time, the entire internet couldn't help but speculate: If this paper is eventually approved through a rigorous peer review, Wang Hong is very likely to become the first Chinese mathematician to win the Fields Medal and the third woman in the world to receive the prestigious award.
The first two women to win were Iranian-American mathematician Maryam Mirzakhani and Ukrainian mathematician Maryna Viazovska.
As the highest honor in the field of mathematics, the Fields Medal is awarded once every four years and is only given to mathematicians under the age of 40.
Referenced author(s): 澎湃新闻·澎湃号·湃客